by Andrew Boyd
Today, one, two, three … divinity. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
What do the following words have in common: natural, imaginary, real, irrational, and transcendental. If you guessed "states of mind," you're probably not alone. But the better answer is they're types of numbers. We take numbers for granted. One, two, three. These are the natural, or whole numbers. What could be simpler? Fractions are no trouble. Half a cup of sugar, a quarter teaspoon of salt. These're called rational numbers because they can be written as the ratio of two whole numbers. But many numbers aren't rational. We call them irrational.
One of the most famous irrational numbers is the square root of two. The Pythagoreans' of ancient Greece discovered this fact. They were a mystic cult, living according to strict rules established by their leader, Pythagoras. Numbers were divine to the Pythagoreans. So when one of their own, Hippasus of Metapontum, discovered the square root of two was irrational, it upset their entire understanding of the world. Legend has it that Hippasus was killed for his heresy. Ironically, we now call the rational and irrational numbers taken together the real numbers. When we add a number called i representing the square root of negative one, we get the imaginary numbers. They're perfectly good numbers, though they'd probably have caused the Pythagoreans to drink hemlock-laced Kool-Aid.
Numbers that consist of both real and imaginary parts – like (3 + 5i) – are called complex.
And things only get stranger. We know that the rational and irrational numbers are both infinite. But there are infinitely more irrationals than rationals. That's because there's more than one type of infinity. This is the result of Georg Cantor's pioneering work on transfinite numbers. Cantor's contemporaries were extremely critical of his efforts. Henri Poincaré called Cantor's transfinite numbers a "disease." Leopold Kronecker, one of Cantor's teachers, called Cantor a "scientific charlatan" and a "corrupter of youth." Today, mathematics and engineering students can't survive without knowing at least some of what Cantor unearthed.
Cantor's work led him to study sets. The set of all red cars, the set of socks in the bottom drawer. Mathematicians believed sets were even more basic than numbers. So in the late nineteenth and early twentieth centuries they went about defining numbers using sets.
It seems crazy. Why fuss with sets? But it laid a foundation that helped us learn many surprising things about what we can and can't do with mathematics. And … with computers.
Today's digital computers work with one thing numbers. Peek inside and you'll see everything expressed in numbers from the icons on your desktop to the email waiting in your inbox. Numbers make our copiers copy, our phones phone, and our word processors process. We couldn't do without them. Wonderful, practical, even divine numbers.
I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.
(Theme music)
| Number | ... | 65 | 66 | 67 | 68 | 69 | 70 | 71 | 72 | 73 | 74 | 75 | ... |
| Character | ... | A | B | C | D | E | F | G | H | I | J | K | ... |
A sample from the very commonly used ASCII (American Standard Code for Information Exchange) chart, showing number/character translations. For example, the letter "capital C" is stored in a computer as the number 67. All information in digital computers is stored in the form of numbers.
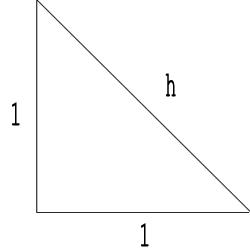
From the Pythagorean Theorem, the Pythagoreans knew that 12 + 12 = h2– that is, h is the square root of 2. They proved that h must be irrational by a simple yet wonderfully ingenious proof, as follows.
If h was indeed rational, then it could be written as the ratio of two whole numbers. Call them a and b, so that h = a/b. We assume a/b is written in lowest terms – that is, there's no whole number c (other than 1) such that a/c and b/c are both whole numbers. (If there were such a number, we'd define a as a/c and b as b/c and work with these new values.)
First, let's show that the number a is even. Since h is the square root of 2, we know 2 = h2 = a2/b2, or 2b2 = a2. Now, the number 2b2 must be even, since it's a multiple of 2 (try it … multiply any number by 2 and convince yourself it must be even). Therefore, a2 must be even (it equals 2b2), and we can conclude that a must be even (this is because a2 is even … if a was odd then a2 would be odd … try it).
Now, let's show that the number b is even. Since a is even, we can write it as a = 2d for some whole number d (the reason for this substitution will become clear in a moment). Look again at the equation 2b2 = a2. Making the substitution a = 2d we arrive at 2b2 = (2d)2, which reduces to b2 = 2d2. Since b2 is a multiple of 2, it must be even, and therefore b must be even.
Since both a2 and b2 are even, they are both divisible by 2. But this contradicts our initial assumption that h = a/b is written in lowest terms. This contradiction proves that no whole numbers a and b exist such that a/b equals the square root of 2.
The logic is undeniable, but the conclusion confounded the Pythagoreans.