Today, we wonder about counting to infinity. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
When I was in grade school, Time magazine ran an article that snatched my imagination. Someone proposed a new number called the googol -- a one followed by a hundred zeros. Later I learned that it wouldn't help very much in counting real objects, because there aren't that many real objects in the whole universe -- even atoms.
But still, we've all wondered where counting ends and infinity begins. And we have good reason for asking about infinity. Every engineering student soon learns that infinity is not just the end of numbers. If we ask how real systems behave when velocity, or time, or force becomes infinite -- if we ask about the character of infinity -- we get unexpected and profoundly useful answers.
Georg Cantor also wondered about infinity. He was born in Russia in 1845. He wanted to become a violinist like his mother. But his father, a worldly merchant, wouldn't have it. When he was seventeen, his father died, and Cantor went on to finish a doctorate in mathematics, in Berlin. He was only twenty-two when he did.
His career didn't last long -- he burned out before he was forty and spent the rest of his life in and out of mental illness. But what he did was spectacularly important, and it arose out of an innocent counting question. He began with an idea we find even in nursery rhymes. Do you remember
1-potato, 2-potato, 3-potato, 4
5-potato, 6-potato, 7-potato, more?
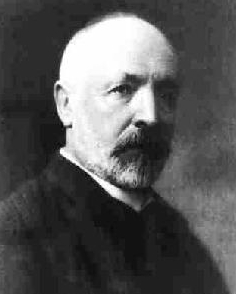 Counting is really matching one set of things with another -- in this case, numbers with potatoes. Cantor asked if counting all the infinite number of points on a line would be like counting all the points in a surface. He identified orders of infinity. Count all the whole numbers and you get the first order of infinity. Raise two to that power and you get the next. And that happens to be the number of sets you can form from the first infinity.
Counting is really matching one set of things with another -- in this case, numbers with potatoes. Cantor asked if counting all the infinite number of points on a line would be like counting all the points in a surface. He identified orders of infinity. Count all the whole numbers and you get the first order of infinity. Raise two to that power and you get the next. And that happens to be the number of sets you can form from the first infinity.
By the time he was done, Cantor had invented what we call the transfinite numbers -- numbers that go beyond infinity. And to do that he'd had to invent set theory. And set theory has become a building block of modern mathematics. Cantor had fallen into an odyssey of the mind -- a journey through a strange land.
Along the way, he had to overcome the doubts of his father, objections of the great mathematicians, and his own doubts as well. When he was 33 he wrote: "The essence of mathematics is freedom." Cantor had to value freedom very highly -- freedom coupled with the iron discipline of mathematics, freedom expressed as the driving curiosity of a bright child, freedom to pursue innocent fascination until it finally touched the world we all live in.
Cantor lived his troubled life until 1918. But that was long enough. He finally saw set theory accepted. He finally saw himself vindicated for that soul-scarring voyage of the mind.
I'm John Lienhard, at the University of Houston, where we're interested in the way inventive minds work.
(Theme music)
Meschkowski, H., Cantor, Georg. Dictionary of Scientific Biography, Vol. III, (C.C. Gillespie, ed.). New York: Charles Scribner's Sons, 1971, pp 52-58.
I am grateful to Professor James Casey, formerly at UH and presently at the University of California at Berkeley, for suggesting the topic.
This is a reworked version of Episode 90.
For more on Cantor, see the website (in German) http://www.mathematik.uni-halle.de/history/cantor/