by Andy Boyd
Today, infinitely befuddling. The University of Houston presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
Imagine a wood block a foot on each side. Now imagine a paper-thin slice cut from the bottom of the block. Here's the question. Which is bigger: the original wood block, or the paper-thin slice?

A wooden block with a thin clice cut from the bottom
It's a silly question - the slice is only a small part of the original block, so of course the block is bigger. But when we look at a mathematical description of the block and slice, the answer's not that simple.
The story begins with Georg Cantor, who upended the world of math at the end of the nineteenth century with his investigations of infinity. Cantor started by asking the following question. How do we know when two sets are of the same size? For example, given a set of cups and a set of saucers, how do we know if there are an equal number of each? Cantor's answer was simple and intuitive: match them up. Set each cup on one saucer. If each saucer has a cup on it and each cup sits on a saucer, we have an equal number of each. If not, one of the two sets is bigger than the other.

Stacked white coffee cups Photo Credit: Pexels
So far this is all quite basic, which is what makes the rest of the story so interesting. Cantor's simple observation leads to some highly counterintuitive results when comparing infinite sets.
Consider the set of all counting numbers 1, 2, 3, 4, and so on, and the set of all even numbers. Both sets are infinite, and our intuition tells us the set of even numbers is smaller because it's a subset of the counting numbers. But under Cantor's definition the two sets are the same size. If the counting numbers are cups, and the even numbers are saucers, we can set cup 1 on saucer 2, cup 2 on saucer 4, cup 3 on saucer 6, and so on. We can match the elements in the sets one-to-one.
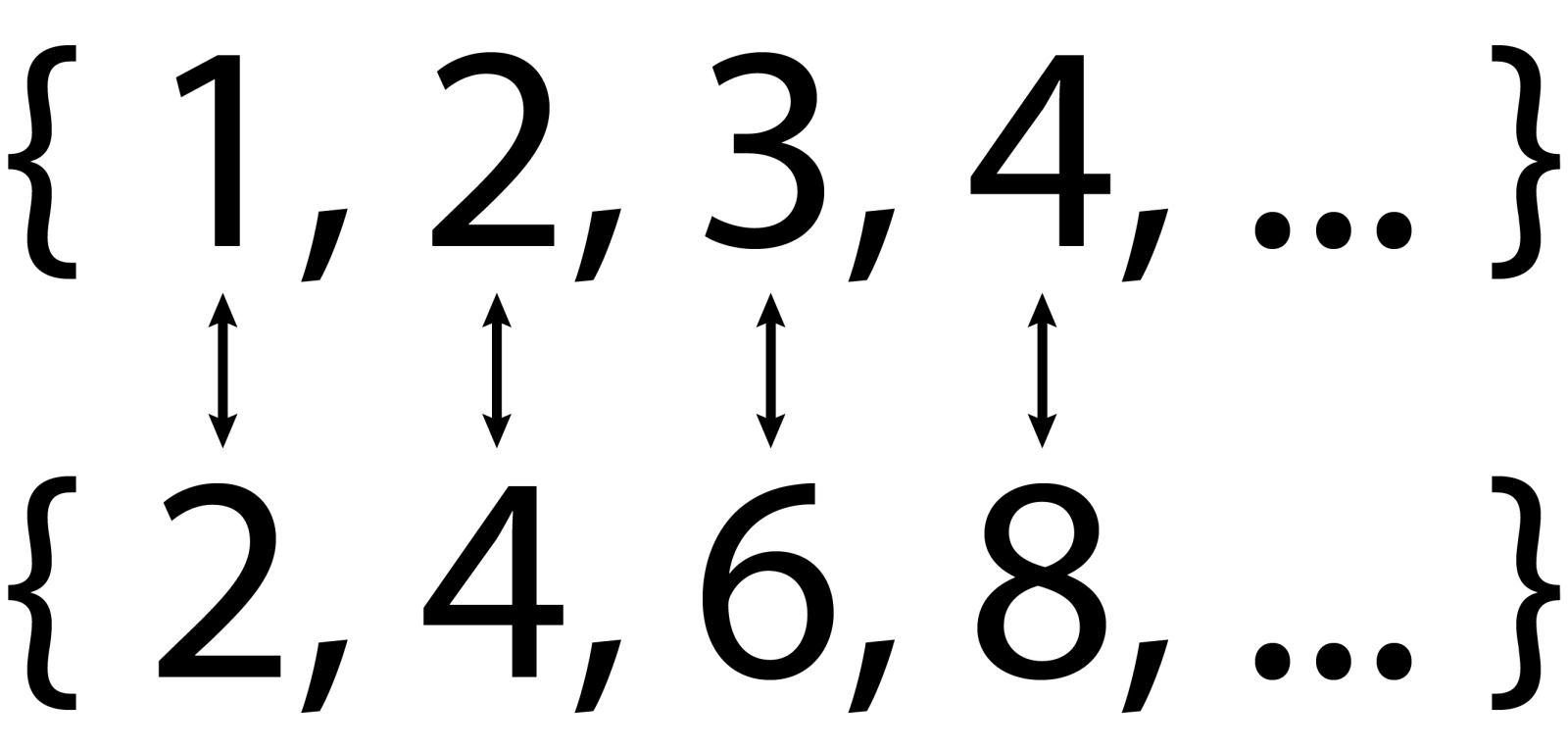
A one-to-one correspondence between the counting numbers and the even numbers
Going back to our block of wood, Cantor compared the set of points in the block with the set of points in the slice. And what he found was astonishing. Even if the slice was infinitely thin, making it two rather than three dimensional, the block and the slice were the same size according to the cup/saucer measure. The points in the block could be put in one-to-one correspondence with the points in the slice.
The result came as a shock to everyone, especially Cantor, who'd spent years trying to prove just the opposite - which both he and other mathematicians thought was intuitively obvious. And the gist of Cantor's proof isn't difficult, requiring little more than an open mind and a willingness to see the logic through. Details can be found on the Engines website.
Cantor had shattered a barrier separating the physical dimensions. Two and three dimensions, and all other dimensions weren't Russian stacking dolls nested neatly inside one another, but Russian dolls seated side by side, hand in hand, even as their relationship remained strangely intertwined. It's yet another example of how perplexing infinity can be.

Russian Matroshka Photo Credit: Wikimedia
I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.
(Theme music)
For clarity, mathematicians use the word cardinality rather than size for Cantor's pairwise measure.
Cantor's original work focused on constructing a two-dimensional square from one of its one-dimensional sides, though the construction is similar for any two dimensions. I have focused on the transition from 2 to 3 dimensions as it seemed more visually evocative for listeners.
Cantor showed that the points in the unit square and the points on the unit line segment could be put into one-to-one correspondence by interlacing points from the square. For example, the point (.364...,.283...) in the unit square would be assigned to the point (.326843...) on the unit line segment. As it stands, this argument needs modification for a technical reason. But the gist of the argument doesn't change. For a more detailed discussion, see Boyd, pages 62-63 and 72.
E. Andrew Boyd. Beyond Comprehension: A Scientific Look at the Challenge of Knowing Everything. Hamilton-Haverbrook, 2017.