Today, simple, yet intricate. The University of Houston presents this series about the machines that make our civilization run, and the people whose ingenuity created them.

In the late 1970s Benoit Mandelbrot caused quite a stir in the world of mathematics. But it wasn't necessarily the kind of stir you want to make.

Mandelbrot was born in Poland in 1924, but moved with his family to France when he was twelve. This situated him in Nazi occupied France during his formative teenage years. Following the war, after a few years of studying and teaching, Mandelbrot made his way to IBM's acclaimed T.J. Watson research center in New York. There he was free to pursue his mathematical interests in a relatively unstructured environment.

Benoit Mandelbrot. Photo Credit: Flickr

It was a golden era as mathematicians were discovering how computers could complement their research. One area of interest was data visualization. While working on a problem related to phone line transmission failures, Mandelbrot looked at a plot of the failures over time. Certain regularities reminded him of something he'd bumped into as a student: the Julia set, discovered by Gaston Julia in 1918.

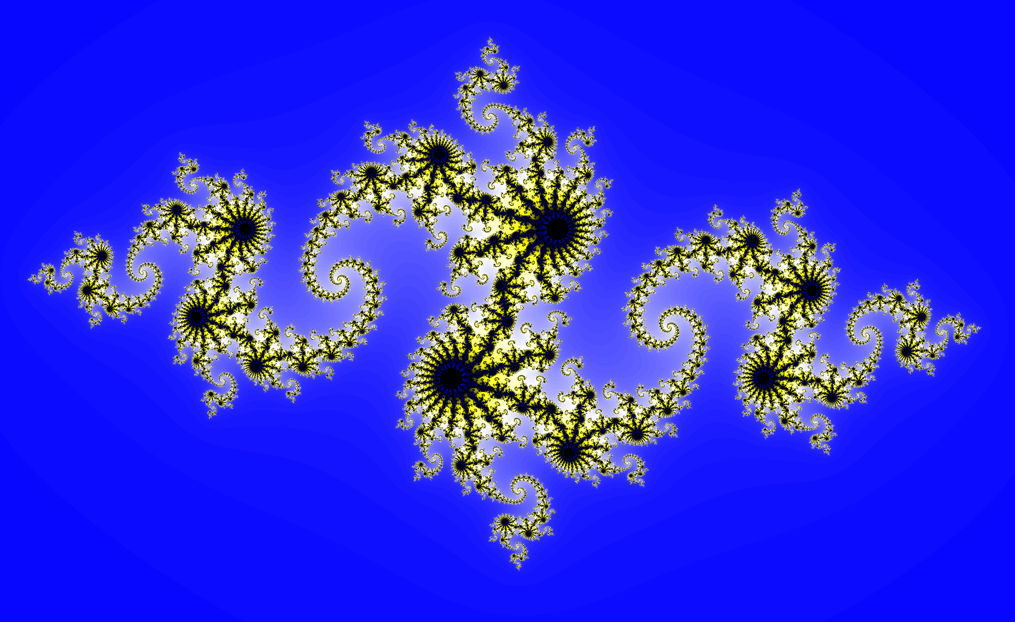
A Julia Set. Photo Credit: Wikimedia

Mandelbrot expanded the notion of the Julia set into something he called fractals. Fractals are sets that have many interesting mathematical properties. For one, they typically have very simple descriptions but take a long time to draw, making the task perfect for computers. They also give rise to incredibly intricate and beautiful shapes. These two properties in particular made fractals ripe for public consumption. At the height of their popularity, a cartoon in the New Yorker magazine depicted a woman who had just redecorated her apartment in fractals.

Fractal table of the collection of Disseny Hub Barcelona. Photo Credit: Wikimedia

Mandelbrot also popularized fractals as something found in nature: the way trees branch, the way clouds form. Today, movie and game animators rely on fractals to generate complicated, real-life images like lava flows and landscapes.

A curliecue leaf. Photo Credit: Pixabay

Leaves show fractal-like properties. Photo Credit: Flickr

A terrain made using Perlin noise. Photo Credit: Wikimedia

Mandelbrot was so instrumental in popularizing fractals that the quintessential fractal is named after him. But as much recognition as he's received, Mandelbrot's work isn't uniformly praised by mathematicians. In part, much of the math is rudimentary, and Mandelbrot never appears to have made much effort to sojourn into deeper realms. It's not clear how novel his contributions were, given the groundwork laid by Julia and others. Even in Mandelbrot's time, others laid claim to priority. And by all accounts, Mandelbrot was quick to accept praise but miserly when dishing it out.

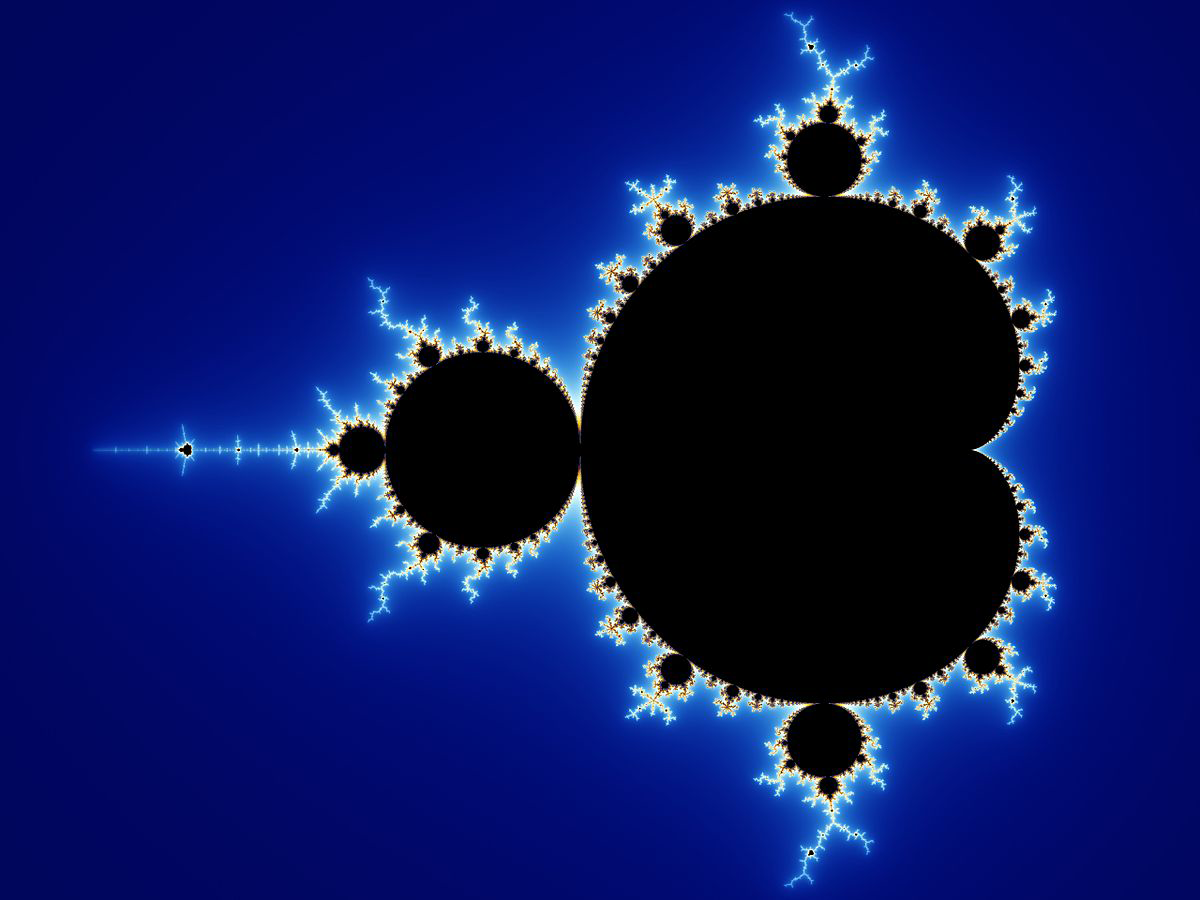
A Mandelbrot set. Photo Credit: Wikimedia

Whatever else may be said, there's no argument Mandelbrot generated awareness of a delightful corner of math to a wide audience. Budding young minds can experiment with fractals on their home computers. Those who are less technically inclined can marvel at the enigmatic beauty of fractals, or spend time searching for fractal structure in the world around them. After all, how often are the complexities of math laid so plainly on display for all to see?

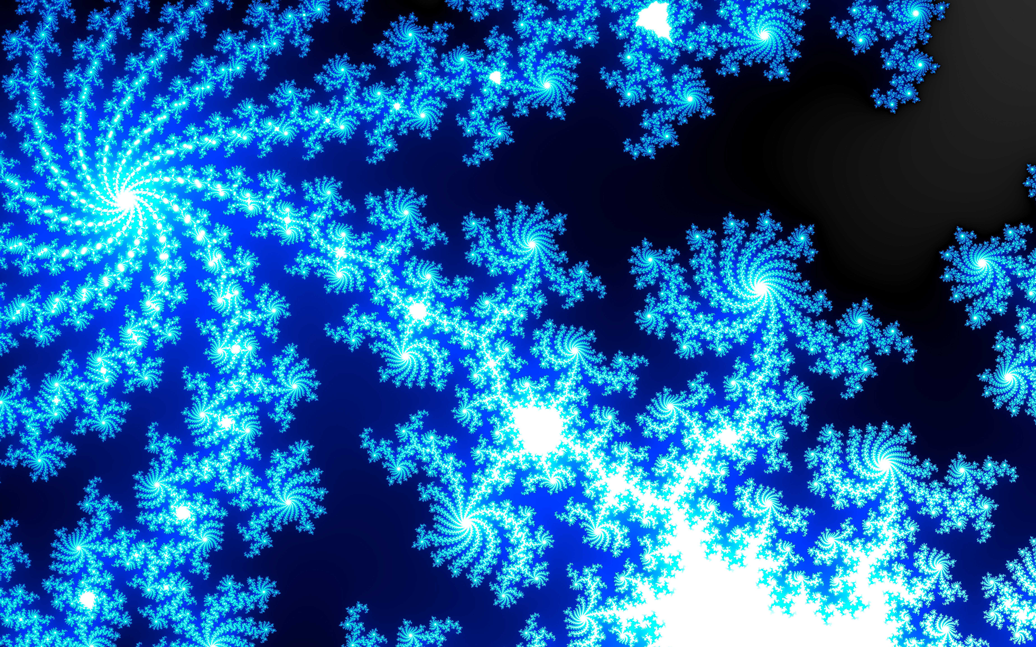
Mandelbrot fractal rendered in Paint. Photo Credit: Wikimedia

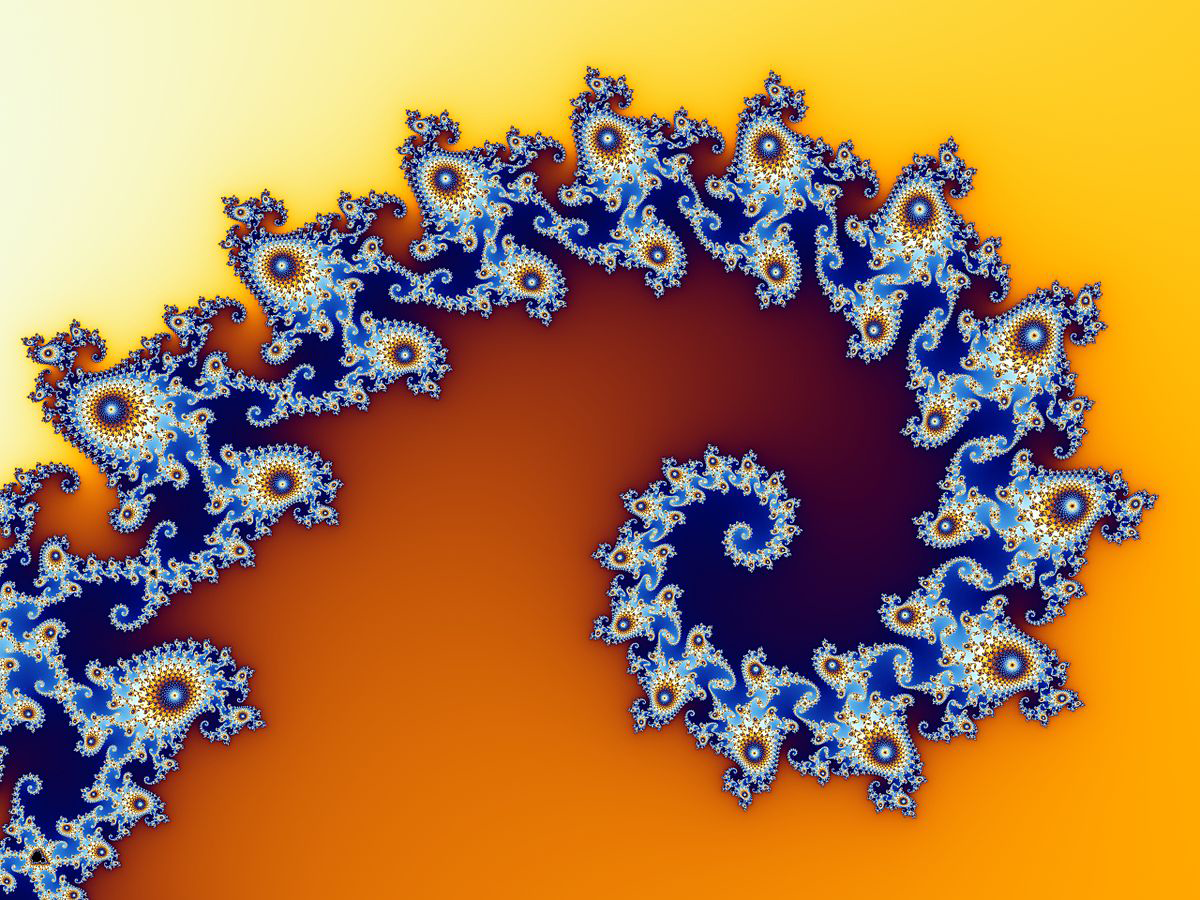
A Mandelbrot set seahorse tail. Photo Credit: Wikimedia

I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.

(Theme music)

---

P. Fatou. "Sur les Substitutions Rationnelles," Comptes Rendus de l'Académie des Sciences de Paris, vol. 164, 1917, pp. 806-808, and vol. 165, pp. 992-995.

J. Horgan. "Who Discovered the Mandelbrot Set? Did the Father of Fractals 'Discover' His Namesake Set?" From the Scientific American website: https://www.scientificamerican.com/article/mandelbrot-set-1990-horgan/. Accessed December 13, 2016.

G. Julia. "Mémoire sur l'Itération des Fonctions Rationnelles." Journal de Mathématiques Pures et Appliquées, vol. 8, 1918, pp. 47-245.

S. Krantz. "Fractal Geometry." Opinion paper in The Mathematical Intelligencer, vol. 11, no. 4, 1899, pp. 12-16.

B. Mandelbrot. "How Long Is the Coast of Britain? Statistical Self-Similarity and Fractional Dimension." Science, New Series, vol. 156, No. 3775. 1967, pp. 636-638.

J. O'Connor and E. Robertson. Benoit Mandelbrot. From the University of Saint Andrews MacTutor History of Mathematics Archive website: http://www-groups.dcs.st-and.ac.uk/~history/Biographies/Mandelbrot.html. Accessed December 13, 2016.

This episode was first aired on December 18, 2016