Today, we get very, very kinky. The University of Houston presents this series about the machines that make our civilization run, and the people whose ingenuity created them.

Henri Poincaré was a renowned nineteenth century mathematician, with contributions spanning many disparate fields. He excelled at math, writing over five hundred scientific papers and thirty books. Yet when it came to math's underpinnings, he was troubled.

Henri Poincaré Photo Credit: Wikimedia

For most of history, math was seen as a reflection of the world around us. Imagine a pile of two apples and another pile of three. Put them together, and you have five apples. Now, as we well know, it's useful to be able to express this process in symbols. Visualize the single-digit characters we use to represent the numbers two, three, and five. Throw in a plus sign and an equals sign, and we can write "2 + 3 = 5." We've used symbols to abstractly express something real.

Poincaré lived in a period when mathematicians were increasingly setting the real world aside and focusing on the formal manipulation of symbols. It's a trend that continues to this day. Algebra started as a means for merchants to solve problems they ran into. Today, algebra in its purest form is defined strictly in terms of symbols and rules for manipulating them.

The formal abstraction of math has many advantages, not the least of which is that nothing's left to interpretation. A mathematical statement is either true or it isn't. There's no ambiguity. But as Poincaré's peers embarked on their formalist agenda, they discovered what Poincaré would refer to as mathematical monsters.

As but one example, imagine for the moment a hose with a kink in it. Now imagine a hose with one hundred kinks. Finally, imagine a hose with kinks everywhere. The hose is nothing but kinks. What does this even mean? If there's a kink, doesn't that mean there's a short stretch of hose on either side of the kink that isn't kinked?

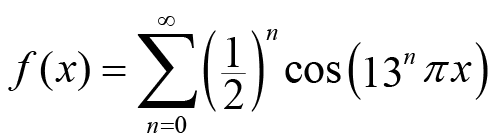
The infinitely kinky (continuous but non-differentiable) Weierstrass function. Observe that even though the function itself isn't smooth, the individual components of the summation are. Photo Credit: Wikimedia

Scientists work with functions, not hoses, but when an infinitely kinky function was first constructed it was confounding. Functions that model our physical world - the orbits of planets, the speed of cars - are kink-free. There's an occasional kink, but nothing to write home about. The idea that a function could be kinky everywhere seemed absurd - and it still does. Yet, as math was made ever more formal and precise, we found we just couldn't escape such oddities.

Today, most students simply laugh when they encounter their first mathematical monsters. But for Poincaré and like-minded mathematicians of his day, the situation was disturbing. Math, they believed, flowed from the human mind, not from rules set down on paper. If the mind couldn't conceive what the rules were saying, something was wrong with the rules. It's a point well taken, but one ignored by most. In the end, we tend to live by the words of another great mathematician, John von Neumann: "In mathematics you don't understand things. You just get used to them."

I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.

(Theme music)

The "kinky" function described in this essay is the Weierstrass function, as described at this Wikipedia web page:  https://en.wikipedia.org/wiki/Weierstrass_function. Accessed June 16, 2017.

William Bragg Ewald. From Kant to Hilbert, Volume 2. Oxford: Oxford University Press, 2007.