Today, infinity. The University of Houston's College of Engineering presents this series about the machines that make our civilization run and about the people whose ingenuity created them.
I'm sure that you, like me, have been unsettled by the idea of infinity. Suppose we have a quantity that increases to infinity. Okay, now take that same quantity, multiply it by three, and let it grow. Is infinity now three times larger? Or square the quantity. Now, as it grows, it becomes infinitely larger than our first infinity. So how many infinities are there!
Of course the problem rises because we're incapable of grasping any physical concept of infinity. We call God, Infinite, for just that reason. Indeed many people see God as a name for that which lies beyond comprehension.
Cosmologists are generally cautious about whether our Universe is finite. It appears to be; but is ours the only Universe? And even it is more than our telescopes and our logic have so-far revealed. Remember that the radius of our 14 billion-year-old Universe now seems to be 46 billion light years. That's because space itself expands much faster than the speed of light.
So we try to wrap our mind around what infinity means in physics as well as in math. We ask, is our physical world ultimately unlimited - and, if so, how? That's a lot like asking what unlimited means in mathematical terms. And, by the way, the mathematicians do lead us on a merry chase!
Late 19th century mathematician Georg Cantor really set our minds awhirl after he laid the foundations of modern set theory. You see, he angered many colleagues when he used set theory to define different kinds of infinities. And now some mathematicians are circulating a really bizarre infinity result on You-Tube. They ask, what's the sum of all the natural numbers, 1 + 2 + 3 and so forth? The result is obviously infinity, right?
Well, by manipulating sums they all-to-easily build a proof that the sum is not infinity, but minus 1/12. That sounds insane. But infinity is a spooky place. What it means to add and subtract out there gets blurry. I'll post that proof on line and let you all take up worrying about it, for I have given up.
Philosophers also get into the game. They object when someone builds an argument on an infinite regress of causes. We can prove almost anything by pulling infinity into an argument (like adding up numbers and getting minus 1/12, I suppose.) That's why the Big Bang theory (with or without God as a first cause) is a lot more acceptable than some infinite regress of cause and effect.
One last thought: While cosmologists, mathematicians, and philosophers deal with their infinities, we engineers also deal with ours. We often have to know what happens when quantities become very large or very small. That means knowing the basic math of competing infinities. Infinity may not be a place we actually go, but, to explain behavior in our finite lives, we must deal with it. Infinity is there and, it seems, we really cannot escape putting it to use.
I'm John Lienhard at the University of Houston, where we're interested in the way inventive minds work.

MIT students call this passage in their main building, "The Infinite Corridor," since it seems to go on forever. It's a nice reminder of the way we create finite analogs in our attempts to visualize infinity. (Next time I'm there, I'll try going to the end to find -1/12.) (Picture by J. Lienhard)
(Theme music)
See the Wikipedia articles on Infinity, Georg Cantor, Universe, and Big Bang,
Click here for the proof that the sum of natural numbers is -1/12 See more on this notion in Episode 2928. See also Episode 2885 about Big Numbers. It alludes to the idea. The -1/12 result is very important in the field of String Theory.
See also the Wikipedia article on L'Hôpital's rule. This is a mathematical way of determining what happens when two quantities approach infinity or 1/zero in such a way as to give a result that does not appear to have a determinate result. (like 0/0 or 0times∞ or ∞/∞) We apply this idea to heat exchanger design in the example below (from Lienhard and Lienhard, A Heat Transfer Textbook.)
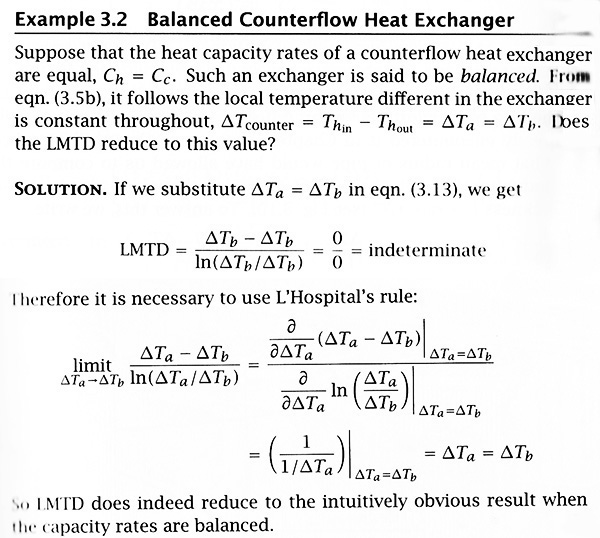
Finally, Engines colleague Kreso Josic points out that a group of mathematicians who call themselves Finitists want to eschew any ac-ceptance of infinite "objects". See more in this Wikipedia page.
This episode was first aired on July 25, 2014