Today, that can't be, can it? The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
In 1703 Guido Grandi caught the world of math by surprise. But before we get to that surprise, let's do an experiment.
Reach out your hand and touch whatever's in front of you. Congratulations. You've just shown it's possible to add together an infinite sequence of numbers and arrive at something finite. Your hand travelled halfway, then halfway again, then halfway again — an infinite number of halfways. Said another way, 1/2 + 1/4 + 1/8... stretching out to infinity equals one.
So adding an infinite sequence of numbers isn't magic. But if we're not careful, strange things can happen. Guido Grandi looked at this very, very simple summation: 1 minus 1 plus 1 minus 1 and so on forever. He then asked what this added up to.
1-1+1-1+1-1...
Taking the numbers in sequence shows they don't add up to anything. One minus 1 is 0, plus 1 is 1, minus 1 is 0, and so on. The sum jumps back and forth between 1 and 0.
But suppose we did our addition a little differently. Let's start by adding the first two terms — 1 minus 1 — that's 0. Now let's add the third and fourth terms — again, that's 1 minus 1, or 0. By pairing terms in this way, everything becomes a zero. And when we add a bunch of 0s — even an infinity of them — we get 0. We've just shown that the infinite sum is actually 0.
Problem is, we can use a similar argument to show the infinite sum is 1. And with just a little more work we can argue it's 1/2. That's right — 1/2. Our algebraic intuition leads us astray.
Grandi was a well regarded mathematician, but he was also a Jesuit. Upon demonstrating the sum was both 0 and 1/2, he proclaimed it showed God could create something from nothing. Mathematicians of the day weren't so moved, but Grandi's work served as a catalyst to find out what was going on. Infinite sums were starting to pop up everywhere during Grandi's time, and they posed a threat to the orderly world of mathematics.
In the end, the solution wasn't overly complicated. Loosely speaking, when an infinite sequence of numbers adds up to something finite we typically don't encounter problems. When it doesn't add up, like jumping from 1 to 0 or the total running off to infinity, seemingly correct arguments are not. One highly celebrated summation is 1 plus 2 plus 3 plus 4 going on forever. The total clearly runs off to infinity. But with nothing more than a little high school algebra we can show it equals -1/12.
At one level, sorting out the devious weeds is just good math. But at another, when the most basic rules of arithmetic fail, it's deeply unsettling. As our dealings with the infinite constantly make us aware, it's tough trying to peer over an edge that isn't there.
I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.
(Theme music)
Notes and references:
History of Grandi's Series. From the Wikipedia website: https://en.wikipedia.org/wiki/History_of_Grandi%27s_series. Accessed February 10, 2014.
Luigi Guido Grandi. From the Saint Andrews website: http://www-history.mcs.st-andrews.ac.uk/Biographies/Grandi.html. Accessed February 10, 2014.
The reader should recall that the 'proofs' given below are fallacious since arithmetic rules are applied in ways that, while perfectly valid for finite sums, are unacceptable for many infinite sums.
Grandi's infinite sum: 1 - 1 + 1 - 1 + 1 - 1 ...
Performing pairwise additions starting with the first term yields:
(1 - 1) + (1 - 1) + (1 - 1) ... = 0 + 0 + 0 + ... = 0
Performing pairwise additions starting with the second term yields:
1 + (-1 + 1) + (-1 + 1) ... = 1 + 0 + 0 + ... = 1
To show that the summation equals 1/2, we recognize that dividing 1 by (1+x) gives us the infinite (power) series
1/(1+x) = 1 - x + x2 - x3 + ...
Evaluated at x = 1 we find
1/2 = 1 - 1 + 1 - 1 ...
The infinite sum 1 + 2 + 3 + 4 + ...
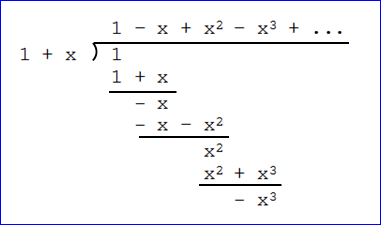
Consider the slightly different sum S = 1 - 2 + 3 - 4 + ...
Add this number to itself, but do so after shifting the second copy to the right by one:
1 - 2 + 3 - 4 + 5 - ...
+ 1 - 2 + 3 - 4 + ...
1 - 1 + 1 - 1 + 1 - ...
So S + S = 2S = 1 - 1 + 1 - 1 + 1 ...
Above, we argued that this series had a value of 1/2 (and many other values ... we adopt 1/2 for the remainder of the discussion).
Thus, 2S = 1/2, or equivalently, S = 1/4 (which is already crazy).
To continue, call our original summation of interest T, so
T = 1 + 2 + 3 + 4 + ..., and calculate T - S:
- 1 - 2 + 3 - 4 + 5 - 6 + ...
4 + 8 + 12 + ...
Notice that this difference can be written as
4(1 + 2 + 3 + 4 + ...) = 4T.
Summarizing, T - S = 4T, or rearranging terms, T = -(1/3)S. But above we argued S = 1/4. Therefore,
T = 1 + 2 + 3 + 4 + ... = -(1/3)(1/4) = -1/12.
The argument presented here is based on an argument found at the website: https://www.youtube.com/watch?v=w-I6XTVZXww. Accessed February 10, 2014.
This episode first aired on February 13, 2014.