by Andrew Boyd
Today, we stick out. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
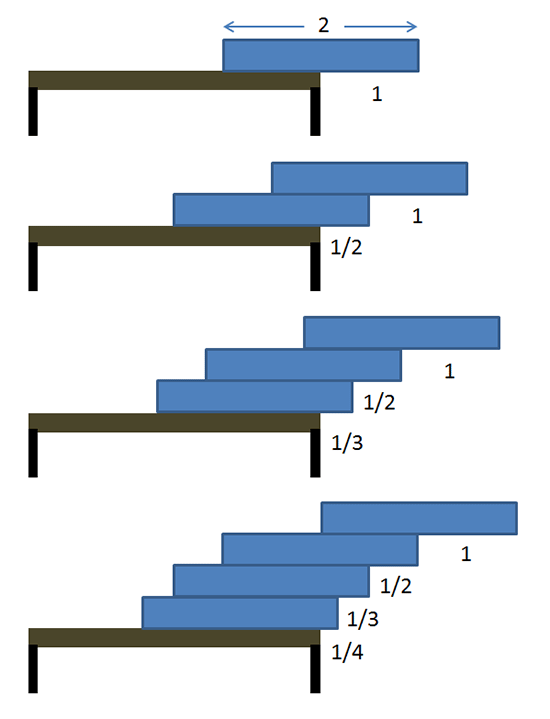
Here's a game you can try at home. Let's call it overhanging books. All you need are some books and a table. For descriptive purposes, I'm going to assume all my books are two feet tall and identical, but you can play with any books on your bookshelf.
If I take one of my two foot tall books and lay it on its side, I can slide it over the edge of the table until it sticks out about half its length, or one foot. Any further and it topples off. That's not an especially exciting observation. So let's take two books, one laid on top of the other, and see what we can do. I'll slide the bottom book out, then slide the top book out a bit further. Done in just the right way, we can get the two book combination to stick out a half foot further than the single book. Try it yourself, or check the Engines website.
It turns out that with every book we add to the stack, we can build the overhang a little bit further. A third book and we could make the overhang 1/3 foot longer; a fourth book, 1/4 foot longer, and so on. The shape of the piled books resembles that of half an arch reaching precariously over the edge of the table. And we're led to ask: at what point does our arch come crashing down?
[Photo by: Andy Boyd]
The answer traces its roots to Nicole Oresme who lived in the late Middle Ages. Bishop of Lisieux, counselor of France's King Charles V — Oresme made contributions in many areas of science and philosophy. While most of the world believed the earth stood still and that the stars and planets revolved around it, Oresme made a case that the heavens stood still while the earth rotated in the middle. Notice that requires Oresme to assume a spherical earth. Oresme didn't make the jump Copernicus would, but then again, Copernicus wouldn't be born for another 150 years.
Through a simple, elegant argument that's still used by math teachers today, Oresme was able to show that 1 plus 1/2 plus 1/3 and so on can be made as large as we want. In the parlance of mathematics, the sum doesn't converge to a finite limit.
So what does this mean for our game of overhanging books? It means that, if we wanted to, we could build a stack of books in the shape of a half arch that overhangs a table by 10 feet or 100 feet or any number we choose. There's no limit on how far we can go.
Of course, it may take a lot of books. A 2 foot overhang can be achieved with only 4 books. Unfortunately, a 4 foot overhang takes 31 books, and a 10 foot overhang requires over 12,000. I don't recommend trying that one at home unless it's a computer simulation.
But notice that we've limited ourselves to one particular arrangement of books — just one on top of another. What if we allowed any stacking arrangement? Now how far and how fast can we build an overhang? It's a question recreational mathematicians have given a lot of thought to. But you don't need to be a mathematician to have fun. Just grab your books and get building. Longest overhang wins'
I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.
(Theme music)
Notes and references:
The sum 1 + 1/2 + 1/3 + 1/4 ... is known as the Harmonic Series and shows up in many different mathematical settings. Oresme's proof that the sum runs off to infinity is as follows:
1 + 1/2 + 1/3 + 1/4 + 1/5 + 1/6 + 1/7 + 1/8 + 1/9 + 1/10 ...
> 1 + 1/2 + 1/4 + 1/4 + 1/8 + 1/8 + 1/8 + 1/8 + 1/16 + 1/16 + ...
> 1 + 1/2 + (1/4 + 1/4) + (1/8 + 1/8 + 1/8 + 1/8) + (1/16 + 1/16 + ...)
= 1 + 1/2 + 1/2 + 1/2 ...
J. Chang. Maximum Overhang, Optimum Reward. From the Microsoft Research website: http://research.microsoft.com/en-us/news/features/peresrobbins-030311.aspx. Accessed February 18, 2014.
The Leaning Tower of Lire. From the website: http://www.mathcs.org/analysis/reals/numser/answers/lire_tower.html. Accessed February 18, 2014. Provides a nice descriptive summary of the overhanging book problem.
Nicole Oresme. From the Stanford Encyclopedia of Philosophy website: http://plato.stanford.edu/entries/nicole-oresme/. Accessed February 18, 2014.
This episode first aired on February 20, 2014.
