by Andrew Boyd
Today, almost twins. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
My wife brought the article to my attention. It had been relegated to the last column of the last page of the Science section of the New York Times. When I read it, I was startled. It was a result sure to shake the world of mathematics. But I understood why it received so little attention in the popular press.
The result itself isn't hard to understand. It's one of many simply stated conjectures about prime numbers. Prime numbers have always been popular with mathematicians. They're numbers that can only be divided evenly by themselves and one. Seven is prime. Divide it by a number other than 1 or 7 and there's a fraction left over. Eight is not prime, because it can be evenly divided by 2. We encounter prime numbers as schoolchildren when we learn to add and subtract fractions.
The problem described in the paper had to do with pairs of prime numbers. If you look at a list of prime numbers you'll quickly notice that every so often two primes are separated by just one even number. Examples are 17 and 19, and 41 and 43. The twin prime conjecture states that these pairs show up forever; no matter how far you go on the list of primes, at some point you'll always bump into a pair of primes separated by a single, even number.

The conjecture is thought to trace back to the ancient Greeks, but its actual origin appears lost in history. Today, it's one of the most famous problems in one of the most famous fields of math: number theory, which studies the properties of whole numbers.
The New York Times article reported that in early 2013, Yitang Zhang, a mathematician at the University of New Hampshire, had come tantalizingly close to proving the twin prime conjecture. He hadn't been able to prove that you could always find pairs of prime numbers separated by a single number, but he had been able to prove you could always find pairs that were separated by no more than, brace yourself, seventy million numbers.
Admittedly, seventy million is a lot larger than 1, and that's a good part of the reason the press had had a hard time making a big to-do about Zhang's result. What makes the result so strikingly important is that seventy million is finite. That's infinitely less than infinity, which is where we stood before Zhang's proof.
And the immediate practical ramifications? Well, there aren't any. While it's true that our technological world depends on math, not all math exists for a purpose. Like great works of art, great works of math serve to awe and inspire. Much is accessible only to a narrow field of experts. But much requires only a clear head and the desire to understand. And comprehending math, at whatever level, is an aspect of creativity unique to that marvelous, flawed, exacerbating animal we call human.
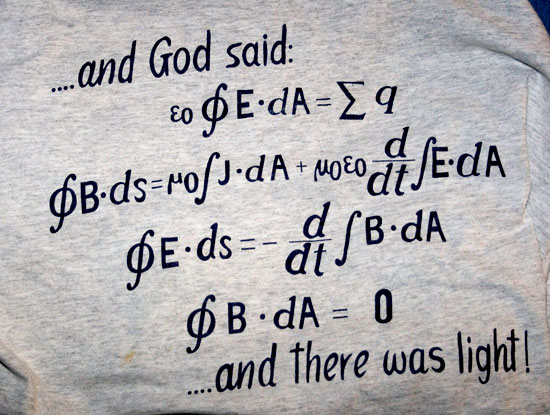
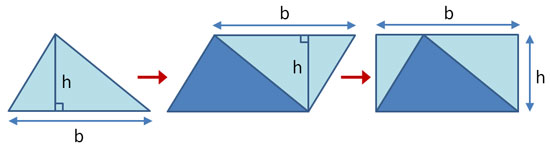
Geometric proof that the area of a triangle is one-half of its base times its height
I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.
(Theme music)
All prime numbers other than 2 must be separated by at least one even number since all even numbers (other than 2) are evenly divisible by 2 and therefore not prime.
For a related episode, see PRIME NUMBERS.
K. Chang. Solving a Riddle of Primes. New York Times, May 20, 2013. See also the New York Times website. Accessed May 29, 2013.
J. Ellenberg. The Beauty of Bounded Gaps. From the Slate website. Accessed May 29, 2013.
The picture of Maxwell's Equations on a T-shirt is from the flickr website. The remaining pictures are by E. A. Boyd.
This episode first aired on June 6, 2013.