by Andrew Boyd
Today, the impossible. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
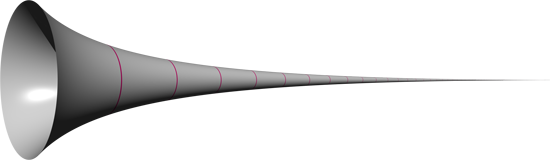
In 1641, Evangelista Torricelli did something that delighted some, shocked others, and made Torricelli famous. He discovered a mathematical figure that stretched to infinity but wasn't infinitely big. Known as Torricelli's trumpet, it's shaped like a long, straight horn. So long that it never ends in a mouthpiece. It gets thinner and thinner, stretching on to infinity. But — and here's the issue — it has finite volume. You can pour in water and even though the horn has no bottom it will get full.
Mathematicians of the day were only beginning to grapple with infinity. As such, the figure was compelling. One leading mathematician responded to a letter from Torricelli writing, "I received your letter while in bed with fever and gout, but in spite of my illness I enjoyed the savory fruits of your mind. And having spoken of [your discovery] to some of my students, they agreed that it seemed truly marvelous and extraordinary.''
But many leading philosophers weren't so thrilled. How could mathematics be trusted if it led to such intuitively ridiculous results? Empiricists like John Locke and Thomas Hobbes believed all knowledge came from what we experience in life. Since we can't experience infinity, they argued, we can't make definitive statements like "Torricelli's infinite horn has finite volume." To connect the finite, which we can experience, with the infinite, which we can't, was folly. Philosophers from all walks found Torricelli's result an enigma. Even Torricelli appreciated the broad repercussions of his work, referring to his discovery as "paradoxical."
Today, we work with infinite figures so regularly they fail to raise an eyebrow. First year calculus students calculate the volume of Torricelli's trumpet as a simple homework exercise. It still remains an object of curiosity, but interestingly, not for the same reasons it challenged great thinkers of the past. For it turns out that not only does Torricelli's trumpet have finite volume, but it has infinite surface area. And as calculus teachers are quick to point out, that means you can fill it with paint, but you can't paint it.

Does that make any sense? Certainly it takes more paint to fill a paint can than it does to paint it. But the situation is reversed for Torricelli's trumpet, and by no small measure. It takes only a finite amount of paint to fill it, but an infinite amount to paint it.
Math students laugh at the paint analogy, then quickly forget as they do their best to keep up with the teacher. And that's a shame. For math is more than a calculating tool. It's a world of great beauty punctuated with surprises. I understand and believe what the math is telling us about Torricelli's trumpet. But it still leaves me smiling as I stand staring toward the infinite.
I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.
(Theme music)
Notes and references:
P. Mancosu and E. Vailati. 1991. "Torricelli's Infinitely Long Solid and its Philosophical Reception in the Seventeenth Century." ISIS, 82:50-70.
Torricelli's Trumpet from Wikipedia.
The picture of Torricelli's trumpet, also known as Gabriel's horn, is from Wikimedia Commons. The picture of the paint cans is from a website of the Alabama Forestry Commission.
This episode was first aired on January 24, 2013.