Today, dimensionless groups. The University of Houston's College of Engineering presents this series about the machines that make our civilization run and about the people whose ingenuity created them.
So what is a dimensionless group? Well, here's one we all recognize: The Mach number -- the ratio of the speed of something compared with the speed of sound. Why dimensionless? It's because we can report speed in many ways -- miles per hour, meters per second, and so forth. Whatever dimensions (or units) we choose, the Mach number will be the same for any situation, for any given airplane, rocket, bullet, or baseball. A Mach number of 2 means something goes twice the speed of sound -- no matter its actual speed or size.
Or try this: The ratio of the circumference of an ellipse to its smallest radius. For any value of that ratio, the ellipse will have the same shape, regardless of its size. And for one special value, it'll always be a circle -- that well-known number is Pi.
So, those are two simple dimensionless groups. Now let's get fancier: Think about a water faucet that's only partly opened. A thin stream of water comes out. It's a solid cylinder of water for several inches. Then it forms into droplets that appear to us only as a blur. That's because surface tension pulls the stream into growing waves. And the waves break the stream into those droplets.
Next, do that with a stream of light oil -- same diameter, same speed. It has much lower surface tension, so it'll go much farther before it breaks up. But, if we shrink the diameter of the stream, or let it emerge more slowly, we can make the break-up look just like the water jet.
This situation has four variables in it: One is speed. But there's also surface tension, liquid density, and the stream's diameter. Nothing as simple as a Mach number or Pi will tell us when the two jets are similar. But we engineers are not deterred.
This time we write a more complicated dimensionless group called the Weber number. It involves all those variables. When the Weber number is the same for two very different liquid streams, they'll break up in the same way.
If we engineers had to do laboratory tests of every situation that ever occurs, we'd never begin building anything. So we have to know when knowledge of one situation can serve another. We do that by expressing our lab results in dimensionless form. That way, we can, say, model airfoil behavior at lower speeds in water. We can model the way gravity affects a large system, by building a small system and putting it in a centrifuge.
Very often, a process depends on so many variables, that we need two, three, or even more, dimensionless groups to write a general description. And, by now, we've created hundreds of such groups. But, once that's done, we can offer one test -- one equation -- that can answer a thousand specific questions.
And all this is far from arcane. Think about using a ten-thousand-to-one scale map or watching a one-twenty-fourth scale model train. What we're really doing is using very familiar dimensionless groups to provide a new perspective upon the world around us.
I'm John Lienhard at the University of Houston, where we're interested in the way inventive minds work.
I say more about the formation and use of dimensionless groups in Section 4.3 of A Heat Transfer Textbook (which you may download in its entirety by clicking here here.) Throughout the rest of this book (or any such book) dimensionless groups are used to generalize data.
For a list of many of the more familiar dimensionless groups see this web site. https://en.wikipedia.org/wiki/Dimensionless_quantity
Click here for a short paper that shows the Weber number being put to use in generalizing an experiment with developing waves on a jet:
And this 1970 NASA Report shows how I used centrifuge to verify the modeling laws that describe the behavior of boiling at other than earth-normal gravity as well as it's similarity to high current density electrolysis.
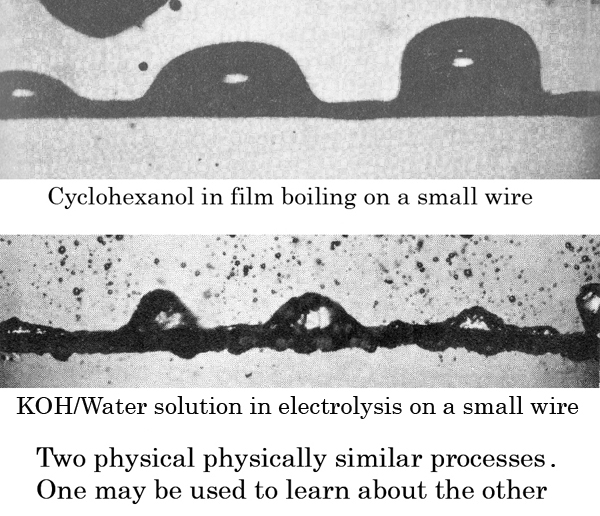
Images: The Electrolysis/Boiling images above are from the preceding NASA report. The falling water jet photos are by John Lienhard.
This episode was first aired on September 4, 2012