Today, I'll bet you never heard of a trumpet squinch. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
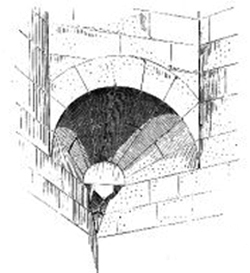 A trumpet squinch is almost as hard to describe as it is to build. It's a conical arch that wells up from the joining of two walls in a masonry building. This lovely complex shape supports the floor above and it forms a small apse in the corner.
A trumpet squinch is almost as hard to describe as it is to build. It's a conical arch that wells up from the joining of two walls in a masonry building. This lovely complex shape supports the floor above and it forms a small apse in the corner.
It also makes the Roman arch seem like a mere two-dimensional stack of stone wedges. But even the Roman arch required each stone be pre-cut in a precise wedge shape. And, while they didn't really need mortar, they usually accommodated a thin, uniform mortar layer exactly a sixteenth of an inch thick.
But cuttings like those squinches take it all into three dimensions -- vastly more complex. From the 10th to 13th centuries, Arabic and European architects began bending masonry into remarkable forms -- complex rib-work on the roofs of vaults, helical stairways, arches intersecting at strange angles.
Architectural historian Sergio Sonabria tells how they did all that without any formal geometry. Geometry was central to medieval scholasticism, but it was the study of logic -- not a means for making things. Yet masonry cried out for geometric technique. Shaping stones to these complex forms is called stereotomy -- which means solid-cutting.
By the 13th century, masons were inventing their own approximate geometry to describe stonecutting. But they didn't write down formal means for making the glorious Gothic cathedral until that era was ending. Then famine, plague, and a retreat from grandeur. A new architecture finally emerged in the 16th century, after printing presses had brought Euclid's geometry to a broader public. Now exact geometrical methods could drive architecture.
Sixteenth-century architects started using their new intellectual apparatus to design magically spatial forms: barrel vaults, biased arches, helicoids, and that trumpet squinch. Just imagine trying to cut the stone blocks that can be piled into such a form. Actually, the late 16th century was giving birth to two new institutions. One was modern mathematical analysis. That wonderfully fluid shape of baroque architecture was the other.

The West portal of National Cathedral in Washington, D.C -- a modern structure, built in accordance with 13th-century masonry. Note the complex three dimensional stereotomy of the cut stone in the arch.
But architectural stereotomy, in its new form as a science, drove design for only a century. Now it gave too much latitude to designers. Mid-17th-century architects went back to the restrictive purity of classical lines, and the people who did mathematical stereotomy became their hired help. The brief, intimate bond between mathematics and architectural design ended; and so too did its hold on our imagination.
Mathematics and architecture went their separate ways for a season. But they met again in the 19th century. Now they gave us such wonders as the Crystal Palace, the Brooklyn Bridge, and the Eiffel Tower. So that trumpet squinch reminds me, yet again, of the way invention is born from ideas that flow separately, then join, and split, and rejoin -- always in new combinations.
I'm John Lienhard, at the University of Houston, where we are interested in the way inventive minds work.
S. L. Sanabria, From Gothic to Renaissance Stereotomy: the Design Methods of Philbert de l'Orme and Alonso de Vandelvira. Technology and Culture, Vol. 30, No. 2, 1989, pp. 266-299.
This is a greatly revised version of Episode 299. The trumpet squinch image above is from the Dictionnaire Raisonne de L'ARCHITECTURE, MDCCCLXVIII. The National Cathedral photos are by J. Lienhard.

Three-dimensional stone cutting in one of the flying butresses of National Cathedral in Washington, DC.
This episode was first aired on October 3, 2011