by Andrew Boyd
Today, the art of attraction. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
Let's play a game. Pick any triangle. Our game creates a sequence of dots inside the triangle. Then we're going to look at the dots we've drawn and see if we can recognize a picture. It's a lot like post-impressionist painting. Look too closely, and you simply see dots. Step back, and something wonderful emerges.
We start by picking any point in the triangle and drawing a dot. Let's call it dot 1. We pick the next dot as follows. Randomly choose one of the triangle's three corners, and draw a dot midway between that corner and dot 1. Then just iterate; that is, randomly pick another corner of the triangle and draw a point that's midway between that corner and dot 2. Call it dot 3. And repeat.
It's a very simple process. But what emerges is stunning. We get a very symmetric, very intricate, very beautiful shape. We start to recognize it after only a few hundred dots. But draw a few million (with the help of a computer) and it's unmistakable. The shape is named after early twentieth century mathematician Waclaw Sierpinski.
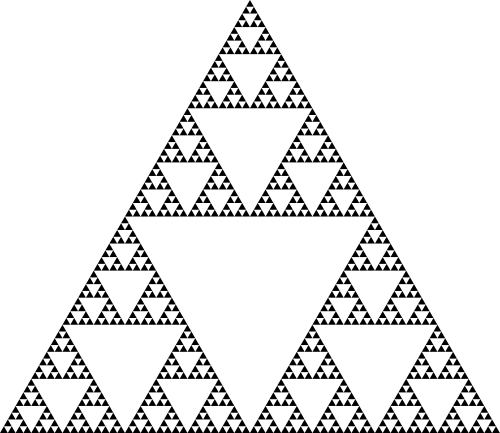
Sierpinski's shape is an example of what mathematicians call an attractor. The rules of our game lead to Sierpinski's shape no matter where we start in the triangle. But we can play many other games with different rules that lead to different shapes. These shapes are called attractors because they pull the points we draw into them no matter where we start. Not all rules lead to attractors, and understanding which rules do is interesting in itself. The fact that the attractors are so alluring is simply one of life's ineffable surprises.
In the book Symmetry in Chaos, authors Field and Golubitsky present us with strikingly graceful figures — in color. Rather than drawing each point with a pencil, they use crayons. If the points are dense in a region, they draw each point with one color. If the points are spread further apart, they use another. Now, rather than seeing intricate shapes, we see intricate shapes within shapes. Swirling lines that could be that could be mistaken for finely woven rugs, except they're far too detailed to be from the hand of any weaver.
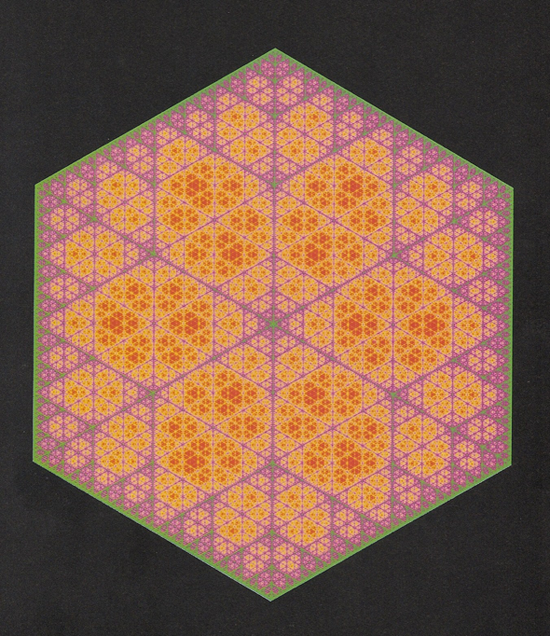
And the most beautiful images are those that come from strange attractors; attractors that arise from games with chaotic rules. Strange attractors provide order in the world of chaos; an order whose breathtaking beauty pulls us in. They're a testament to the extraordinary and perplexing congruity we find in so many corners of our universe.
I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.
Notes and references:

For a related episode, see 1541, HISTORY AND HORSESHOE NAILS.
The Sierpinski shape is commonly referred to as the Sierpinski Triangle. Here, I have used the term "shape" to refer to the attractor itself — the region colored black within the triangle — to avoid confusion.
When playing the dot game, more commonly known as the chaos game (see the Chaos Game reference), some of the first few points may not be on the Sierpinski shape. However, the points typically move into the Sierpinski shape relatively quickly.
Beyond the dot game, the Sierpinski shape can be constructed using the method suggested by the sequence of pictures of approximations of the Sierpinski shape — placing one "upside down" triangle in the middle of each black triangle at each step. The construction goes on infinitely.
The book by Field and Golubitsky first introduced me to the "art of attraction." Many thanks to then-colleague Marty Golubitsky for his gift. Many pictures of strange attractors can now be found on a variety of web sites.
Chaos Game. From the Wikipedia Web site https://en.wikipedia.org/wiki/Chaos_game. Accessed April 13, 2010.
M Field and M. Golubitsky. Symmetry in Chaos: A Search for Pattern in Mathematics, Art and Nature. Oxford: Oxford University Press, 1992.
The picture of the hexagon, known as the Sierpinski Hexagon, is a realization from the book by Field and Golubitsky. The pictures of the Sierpinski shape are from Wikimedia Commons.