Today, UH Math Professor Krešo Josić talks about music and mathematics. The University of Houston presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
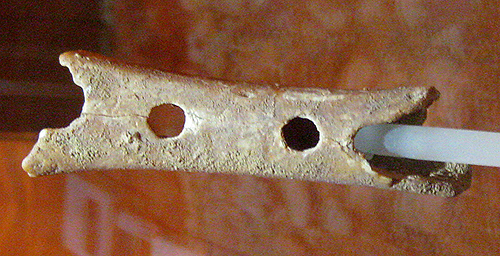
The history of musical instruments goes back tens of thousands of years. Fragments of bone flutes have been found at Neanderthal sites. Early instruments show that humans have long produced pitched sound — sound containing predominantly a single frequency. Finger holes on ancient flutes indicate that prehistoric musicians had some concept of a musical scale.
Music is also the most mathematical art. Already the Pythagoreans of ancient Greece studied the mathematics of musical instruments. According to legend, Pythagoras was listening to blacksmiths at work. He noticed that some of their hammers produced a pleasing combination of sounds when struck together. When he examined these hammers he found that one weighed 12 pounds and another 6 pounds. The first hammer weighed exactly twice as much as the second.

Pythagoras had discovered a fundamental principle of harmony. Most Western instruments produce sound by causing air to vibrate a certain number of times per second. When two instruments cause air to vibrate at frequencies whose ratios form simple fractions — 2:1 or 3:2, say — we perceive a pleasing combination of sounds. The consonance between the blacksmith's hammers caught Pythagoras' attention. For instance, the 6 pound hammer produced vibrations exactly twice as fast as those of the 12 pound hammer. This two-to-one relationship between notes is called an octave, and appears in nearly all music produced by humankind.
But, the problem of translating Pythagoras' observations to the design a musical instruments has bedeviled some of the brightest minds of millennia past. How do we tune a piano, or any ensemble instrument, so it sounds good when it's played with other instruments?

Along with the principles of harmony, Pythagoreans also recognized a primary problem of instrument design. The notes used in Western music can be obtained by starting with a frequency and multiplying it by 3/2, and then by 3/2 again and again. This produces a sequence of notes - spanning different octaves - called the circle of fifths. All notes in the Western chromatic scale can be obtained this way — but only approximately. The problem is that if we keep multiplying a frequency by 3/2, we never get frequencies that are exactly separated by octaves.
Hundreds of different tunings have been proposed to work around this problem. Bach wrote his Well-Tempered Clavier to demonstrate the advantage of a slightly imperfect tuning he favored. With past tunings some music sounded very pleasing, while other compositions could sound woefully out of tune. In nearly all music today we use equal temperament — a compromise that effectively makes all our music sound equally pleasing, or equally out of tune – depending on your perspective.
The next time you consider the cold rationality of mathematics, also remember that math is behind the sound of violins, pianos, and song. The beauty of mathematics shines through in the music it helps us create.
I'm Krešimir Josić, at the University of Houston, where we're interested in the way inventive minds work.
Numerous websites explain the basics of harmony. Here is a very simple explanation that includes an example with sound http://www.aboutscotland.co.uk/harmony/prop.html.
Although idiosyncratic, I like Bill Sethares' approach to tuning. He shows how modern technology allows us to easily create scales with any number of notes. There are some pretty interesting examples on his webpage http://eceserv0.ece.wisc.edu/~sethares/.
The story of the Neanderthal flute has been discussed previously in this series Episode 1232. However, the finding remains very controversial https://en.wikipedia.org/wiki/Divje_Babe_flute.
All images are in the Creative Commons.