Today, exponential growth. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.

A tricky thing about radio is how the goals of being accurate, and being understood, can conflict. It's all too easy to give an illusion of understanding, and to be wholly inaccurate. Example: I drive home, listening to a story about the great George Mason basketball upset of number-one Connecticut. Someone at George Mason is telling about 300 congratulatory phone calls the next day, "and that number," he intones, "is rising exponentially!"

That seems to make sense, but does it? To get exponential growth, we multiply the size by some number in each time increment. If we multiply by two, and the time increment's one day, then he'll get 600 phone calls tomorrow, then 1200, then 2400. That's very precipitous! But suppose the increase is only ten percent per week. Next week we multiply by 1.1 and get 330 -- then 363. That's still exponential, but no longer impressive.

Another common kind of growth is called linear. That means the size increases by the same amount in each time increment -- say a hundred a day. Then we'd get 400 calls tomorrow, 500 the following day, and so on. Linear growth can actually start off a lot steeper than exponential growth. But, given enough time, exponential growth always overtakes it. 

Exponential has become a new drama word, so its real meaning gets lost. And, when that happens, we lose some of our ability to speak accurately. Try another word:

I might say that 19th-century hunting decimated the American buffalo. Alas, that is not the case. To decimate is to reduce by ten percent. We far outran decimation; we came this close to eliminating the buffalo. Yet that might actually have occurred by successive decimations. Ten percent one year, ten percent of the remaining buffalo the next, and so on until the buffalo were almost gone and hunting then became too difficult. (If we do the arithmetic, we find that successive decimations result in what we call an exponential decrease.)

Try another case: Graphs of global temperature show it rising sharply to an all-time high, over the last century. One hears talk of an exponential temperature increase, but one could just as well approximate the jagged increase as linear. What's important is that we not let it rise another full Centigrade degree beyond its present level. For that's the point at which most experts expect irreversible changes that could be devastating. Meantime, the physical processes that cause Earth's temperature to rise are far too complex to describe as either linear or exponential.

Maybe the time's come to go back and revisit our eighth-grade math class -- that world where words like linear, logarithmic, and exponential once had real meanings. Perhaps if we again take such meanings seriously, we'll have better be able to understand data like those that describe real changes afoot in this world that sustains us.

I'm John Lienhard, at the University of Houston, where we're interested in the way inventive minds work.

My thanks to Lewis Wheeler, UH Mech. Engr. Dept., for suggesting the topic.

Listener Stephen Schwartz reminds me that, for just an instant, all exponential growth in time may be approximated as an initial value plus a constant times time. That means that all exponential growth looks linear at first.

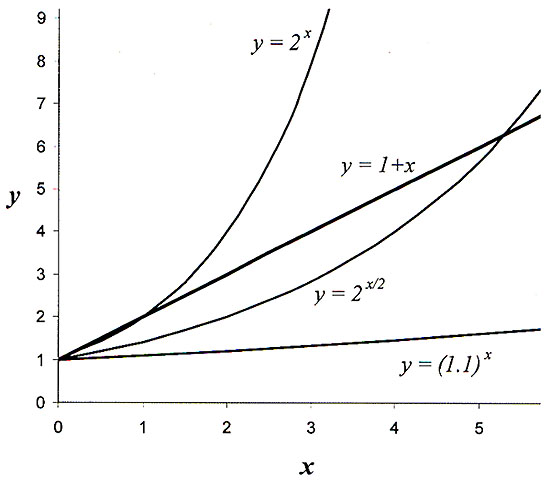
Three examples of exponential growth compared with one example of linear growth