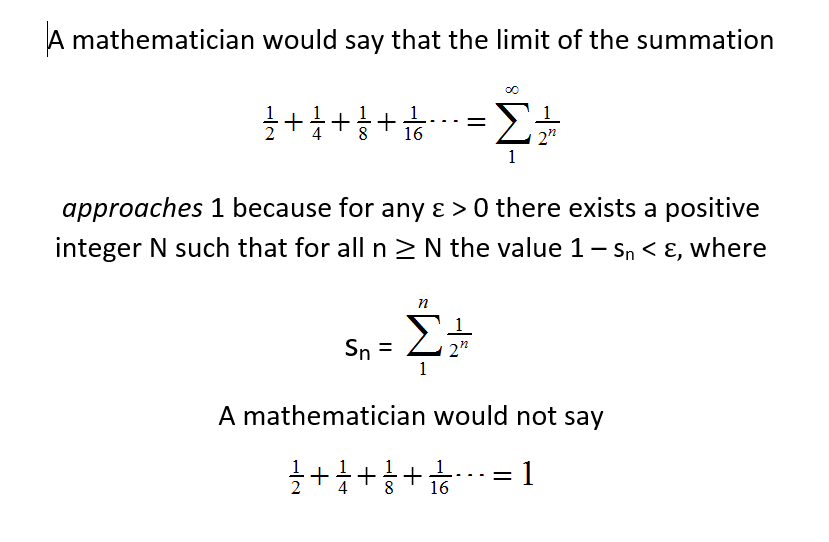Today, seeing and getting. The University of Houston presents this series about the machines that make our civilization run, and the people whose ingenuity created them.

Imagine standing at a traffic light. A car crosses the intersection and you see it smoothly glide through every point along the way. Or do you?

Let's compare how you see with how a camera operates. A typical camera might capture thirty pictures, or frames, per second, and when we play the film back what we see looks continuous. Is it possible our eyes and brain work more like a movie camera? Do we see a sequence of images sequentially pasted together rather than a continuous flow of images? 

Charlie Chaplan on a continuing film reel. Photo Credit: Pixabay

Consider the following experiment. Suppose we try to make a film look more realistic by increasing the number of frames-per-second from thirty to something bigger. Does it work? 

Yes, but only to a point, and with some caveats. When Peter Jackson filmed The Hobbit at twice the frame rate of common theatrical releases, many people didn't notice a difference. Among those who did, many found the higher frame rate looked somewhat unnatural.

Now consider the computational requirements associated with viewing a film on a computer. Doubling the frame rate doubles the number of images per second, which in turn requires the computer to work twice as fast. We can only double the number of frames so many times before the playback speed breaks the laws of physics. And those laws don't just hold for computers. They also hold for the human eyes and brain. That continuously smooth motion of the car you watched? You couldn't possibly have processed every single instant.

The analogy with filming is far too simple to explain how we see. But however our eyes and brain work their magic, they create the perception of continuous motion without processing every moment of action.

Still, independent of how we see, the car itself surely glides continuously through the intersection. Well, let's think about that. Zeno of Elea pointed out that to cross the intersection, an object must first go halfway, then half the remaining distance, then half that remaining distance ad infinitum. How, Zeno asked, can anything reach the other side when it must pass through an infinite number of distances? Interestingly, Zeno's paradox has never been adequately resolved. Ask a mathematician and she'll tell you we can move an object as close as we want to the other side, but mathematical formalism forces her to remain silent on whether it actually gets there. Many philosophers have weighed in over the years, including such luminaries as Bertrand Russell, but there's no final verdict.

Photo Credit: Andy Boyd

Quantum theory provides yet another wrinkle on how the car moves. Energy and motion, according to the theory, aren't continuous, but choppy. Much like the frames of a film playing in a projector, the world jumps from picture to picture with nothing to fill in the gaps. And it all leaves us to scratch our heads and wonder both what we see and what we get.

I'm Andy Boyd at the University of Houston, where we're interested in the way inventive minds work.

(Theme music)

Listeners familiar with modern video formats know that by using video compression techniques a file containing a movie need not double in size even if the number of frames is doubled. However, typical uncompressed formats, where frames are stored individually, do have the doubling property, and it's this frame-by-frame model that's being addressed in the essay. Listeners who are so inclined are free to speculate on whether compressed video provides a better model of human vision.

Reasons why higher frame rates aren't uniformly viewed as better have led to a great deal of speculation about video and vision. For an interesting discussion surrounding the release of The Hobbit, see the following two articles. Note that films are typically released to theatres at a rate of 24 frames per second, making 48 frames per second a doubling for theatres.

J. Fox. What Critics are Saying About The Hobbit's High Frame Rate. From The Vulture website. Accessed June 28, 2016.

See Also: Zeno's Paradoxes. From the Wikipedia website. Accessed June 28, 2016.

This episode was first aired on June 30, 2016