Today, let's talk about models. The University of Houston Mathematics Department presents this program about the machines that make our civilization run, and the people whose ingenuity created them.
The world around is incredibly complex. Even the smallest events have a multitude of causes and consequences. Yet, this does not seem to bother us. How do we make our way through the tangle of interrelated events around us? Consider how we plan our drive to work in the morning. We think about how much gas we have in the tank. However, we do not do a mental check of all the engine parts. We may plan for road closures. But we don't spend much time considering a massive breakout of zoo animals. We use experience and instinct to consider only the most relevant factors. In our minds the commute is stripped of everything unlikely and irrelevant. The result is an image of a world that we can easily navigate in our head.
This seems too obvious to deserve mention. But it is actually quite amazing: Seemingly without effort we get to the essence of what is important. We naturally create models of the complex reality around us, and use these models to guide our actions.
Models are also essential in science. In medicine animal models are used to find and test cures. Physicists use mathematical models to describe the behavior of subatomic particles and galaxies.
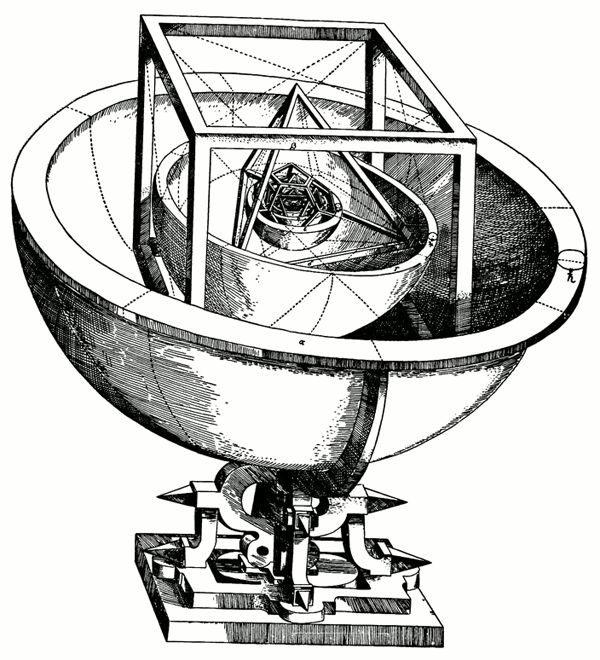
Kepler's Platonic solid model of the solar system from Mysterium Cosmographicum (1596)
However, we need to be very careful. The statistician George Box warned that 'All models are wrong, but some are useful.' Every model is only an approximation. They are only shadows of reality. They are wrong — no shadow captures all the complexities of the real thing. However, stripped of distracting hues and facets such shadows are easier to manipulate in our mind. Models allow us to make sense of the world.
Our innate ability to develop models is excellent, but it is limited to the world we experience with our senses. Mathematics allows us to venture far beyond: Using the language of mathematics we can develop remarkably precise, yet universally comprehensible models — ones that we can share with other humans, and with computers. Using mathematics we can describe the motion of planets and stars, as well as the interactions of genes and proteins. I can't think of a natural process which we will not be able to model using mathematics.
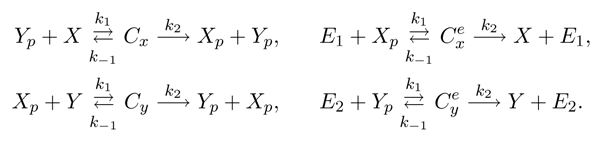
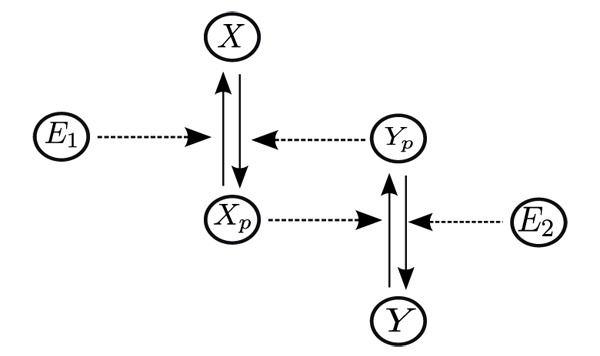
Mathematical model of the mutual phosphorylation of two proteins
It is surprising that the world in all its intricacies is understandable. And it is amazing that models actually work. Why is it that we can take this immensely complicated universe, and understand its behavior with the limited computational power between our ears? Perhaps even more surprising is that we have mathematics — a tool that allows us to create astoundingly precise models of the world far beyond our senses. I am not alone in this sentiment — in the words of the Nobel Laureate Eugene Wigner: "The miracle of the appropriateness of the language of mathematics for the formulation of the laws of physics is a wonderful gift, which we neither understand nor deserve. We should be grateful for it and hope that it will remain valid in [the] future..."
I'm Krešimir Josić, at the University of Houston, where we're interested in the way inventive minds work.
Click here for Wikipedia article about Wigner's essay 'The Unreasonable Effectiveness of Mathematics in the Natural Sciences' with a link to the actual essay.
The Kepler's Platonic solid model of the Solar System from Mysterium Cosmographicum(1596) is from Wikipedia. The other images are by Krešimir Josić.