Today, proof and belief. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
Two recent encounters have left me thinking about proof and belief: an article on proof and public perception in the New York Times, and a tiny book by Burkard Polster, Q.E.D., Beauty in Mathematical Proof. It sets out to show the many faces of mathematical proof.
The Times article points out how, as more scientific questions reach the courts, proof takes on different meanings. In civil law, we ask what the preponderance of the evidence says. In a criminal court, something has to be true beyond reasonable doubt. So criminal courts reminds us of observational scientists amassing enough evidence to make the likelihood of anything else negligible.
Yet a vast gulf separates the attorney and the scientist: The attorney gathers facts to support a claim; while the scientist shapes the claim to match the facts.
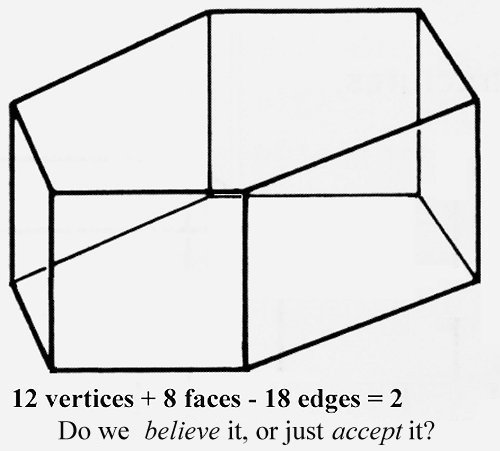
For Polster, proof means demonstrating by logic the absolute necessity that something's true. He talks about proof by dissection, induction, contradiction, regrouping, and so on. He lays out the exquisite simple beauty of mathematical proof. He proves, for example, that the sum of vertices and faces in a diamond, minus the number of edges, is always two. Think about that for a moment:
Can I say that I believe that's true of diamonds? I certainly accept it. But, if I were to see a counter-example, or discover a flaw in the reasoning, I'd relinquish that theorem without a whimper. For, it turns out, proof and belief are oddly disconnected.
Like you, I believe many things. I believed the law of gravity long before anyone told it to me -- I learned at a very early age not to fall out of bed. I believe that God is in the midst of the whirlwind. I believe in people's innate goodness, and in the efficacy of apple pie. But I don't come to any of these beliefs as the result of proof. Belief is our complex integration of a vastly greater sea of evidence than memory or logic can handle.
The word proof takes its worst beating when it's attempted in support of religious beliefs. The medieval church, the Platonist church, strived to build logical proofs of God's existence. Since then, every one of those proofs has folded under close scrutiny.
Today, those same people turn to empirical proofs -- the idea that the world is too complex to've just happened, therefore God created it. Those arguments from design can't be refuted because they can coexist with natural mechanisms. Of course they become dangerous when people expect them to replace natural mechanisms.
Yet, I don't know anyone who was ever convinced of God's existence through proof. Proof gives us, not belief, but confidence in what we all knew or suspected to be true. Or it might tell us when we were wrong in our suppositions.
Proof may not instill belief, but we've missed something very important if we don't understand the value, the structure, and the limitations of proof. I guess that's why I find Polster's brief little book on proof so very appealing.
I'm John Lienhard, at the University of Houston, where we're interested in the way inventive minds work.
C. Dean, When Questions of Science Come to a Courtroom, Truth Has Many Faces. New York Times, Science Times, Dec 5, 2006.
B. Polster, Q.E.D.: Beauty in Mathematical Proof. (New York: Walker Publishing Co., 2006).