Today, let's think about fives. The University of Houston's College of Engineering presents this series about the machines that make our civilization run, and the people whose ingenuity created them.
We bipedal people are made up of two similar left/right halves, and we do love symmetry. We balance our designs and our architecture into complex symmetries and partial symmetries. We like to play with three-dimensional symmetry. A cube, for example, has nine different planes of symmetry and, if you want some fun, you might try figuring out what they are.
The concept of symmetry can be confusing. Hand a person a triangle, a square, and a pentagon, and ask which is least symmetrical. Three out of four people pick the pentagon, even though its five axes of symmetry are the largest of the set. The pentagon, with all that symmetry, doesn't strike us as symmetrical at all.
But its symmetry does play on our subconscious. What fun it is when children learn to draw five-pointed stars with five pencil strokes -- so easy, yet so subtle! And a small pentagon sits in its center. Leonardo da Vinci's spread-eagled human inscribed in a pentagon has become a major icon just because it's so unexpected. Add our head to our arms and legs and you and I become pentagons.
Nature offers so much pentagonal symmetry: the armor of pineapples, cross sections of apples -- starfish, flowers, sand dollars. But we also force five-fold symmetry on many things. The Greeks spoke of five Platonic solids: cubes, octahedrons, tetrahedrons, icosahedrons, and dodecahedrons. The first four (formed from triangles or squares) stood for earth, air, fire, and water. But the fifth, the dodecahedron (made from pentagons) was the symbol for pure celestial matter. That's where the movie The Fifth Element gets its title. Hindu philosophers added celestial ether to their four earthly elements, and they also got the mystical number, five.
Ask musicians how they feel a five-beat measure. They'll tell you, "Twos and threes, Ya-ta Ta-ta-ta, or Ya-ta-ta Ta-ta." We have trouble hearing a full five-beat sequence because the number five seems to lack symmetry in time as well as in space.
Now Istvan Hargittai offers a book of essays, Fivefold Symmetry. Twenty-nine authors hold the idea up to the light and look at its refractions. They find fives lurking everywhere in both nature and iconography. Small wonder that, when Mephistopheles tried to leave Faust, he tripped upon, a "Thin goblin-foot upon the threshold there." Faust had painted a pentagram on the floor and the Devil had to struggle to get around it.
Fivefold symmetry is shot through tile making, architecture, basket weaving, fortifications, and Christian, Islamic, and Egyptian iconography. Fives seem to rise like mist out of our subconscious sense of balance. They're almost never in our mental forefront. Rather, the imagery of fives is one of those odd driving forces within us -- and one it might pay to be more aware of.
I'm John Lienhard, at the University of Houston, where we're interested in the way inventive minds work.
(Theme music)
Hargittai, I., Fivefold Symmetry Singapore: World Scientific, 1992.
I am grateful to my colleagues Lewis Wheeler and N. Shamsundar for their help with this episode. For a fine explanation of polyhedrons and the Platonic solids, you should try the following web site: http://www.georgehart.com/virtual-polyhedra/platonic-info.html.
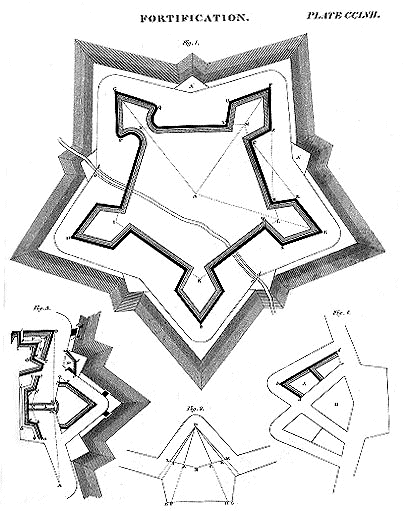
A Typical Early 19th-Century Pentagonal Fortification
From the 1832 Edinburgh Encyclopaedia